A Course in Complex Analysis explores a central branch of mathematical analysis, with broad applications in mathematics and other fields such as physics and engineering. Ideally designed for a year-long graduate course on complex analysis and based on nearly twenty years of classroom lectures, this modern and comprehensive textbook is equally suited for independent study or as a reference for more experienced scholars.
Saeed Zakeri guides the reader through a journey that highlights the topological and geometric themes of complex analysis and provides a solid foundation for more advanced studies, particularly in Riemann surfaces, conformal geometry, and dynamics. He presents all the main topics of classical theory in great depth and blends them seamlessly with many elegant developments that are not commonly found in textbooks at this level. They include the dynamics of Möbius transformations, Schlicht functions and distortion theorems, boundary behavior of conformal and harmonic maps, analytic arcs and the general reflection principle, Hausdorff dimension and holomorphic removability, a multifaceted approach to the theorems of Picard and Montel, Zalcman’s rescaling theorem, conformal metrics and Ahlfors’s generalization of the Schwarz lemma, holomorphic branched coverings, geometry of the modular group, and the uniformization theorem for spherical domains.
Written with exceptional clarity and insightful style, A Course in Complex Analysis is accessible to beginning graduate students and advanced undergraduates with some background knowledge of analysis and topology. Zakeri includes more than 350 problems, with problem sets at the end of each chapter, along with numerous carefully selected examples. This well-organized and richly illustrated book is peppered throughout with marginal notes of historical and expository value.
Presenting a wealth of material in a single volume, A Course in Complex Analysis will be a valuable resource for students and working mathematicians.
Saeed Zakeri is professor of mathematics at Queens College and the Graduate Center, City University of New York. He is the author of Rotation Sets and Complex Dynamics.
- Preface
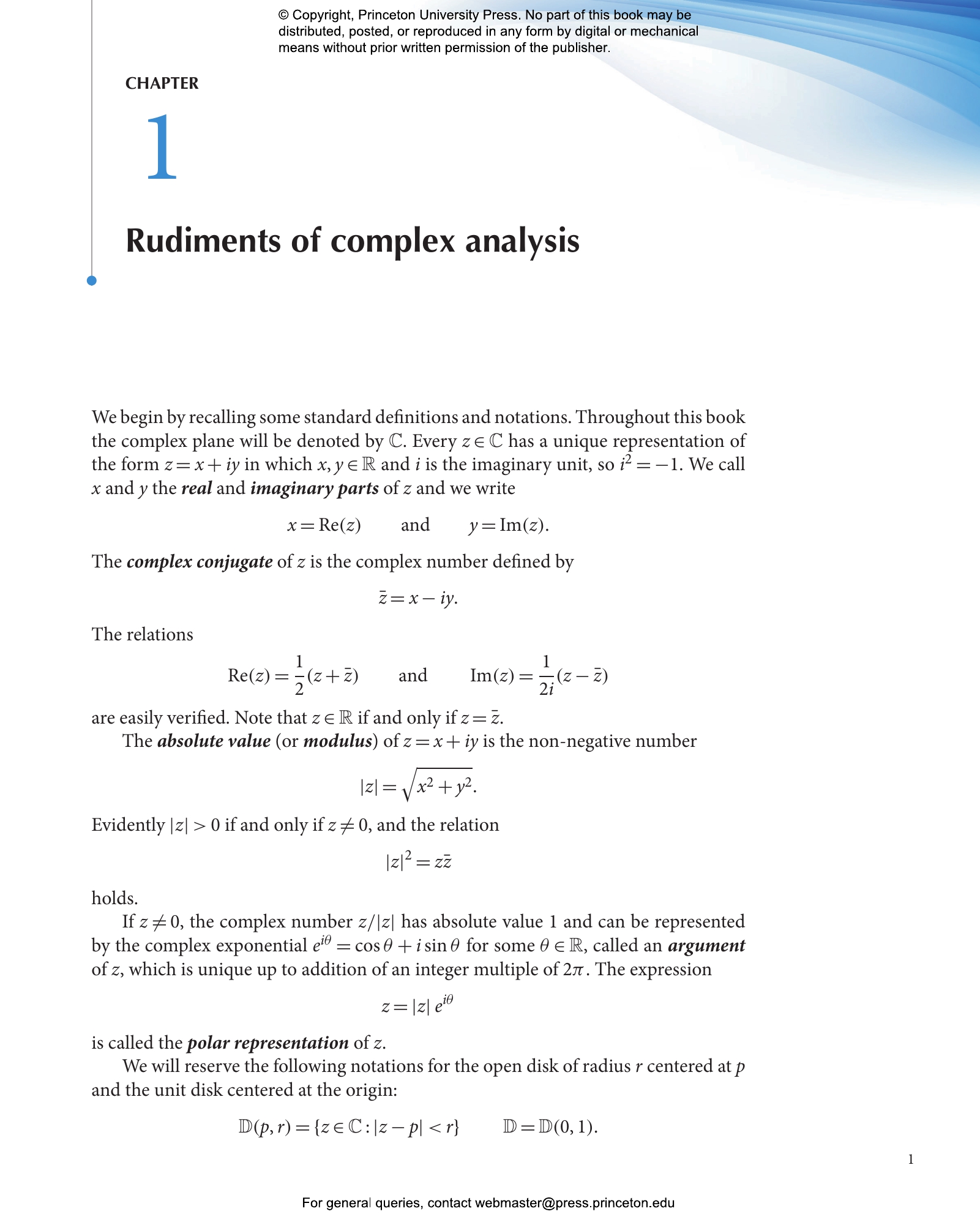
- Chapter 1. Rudiments of complex analysis
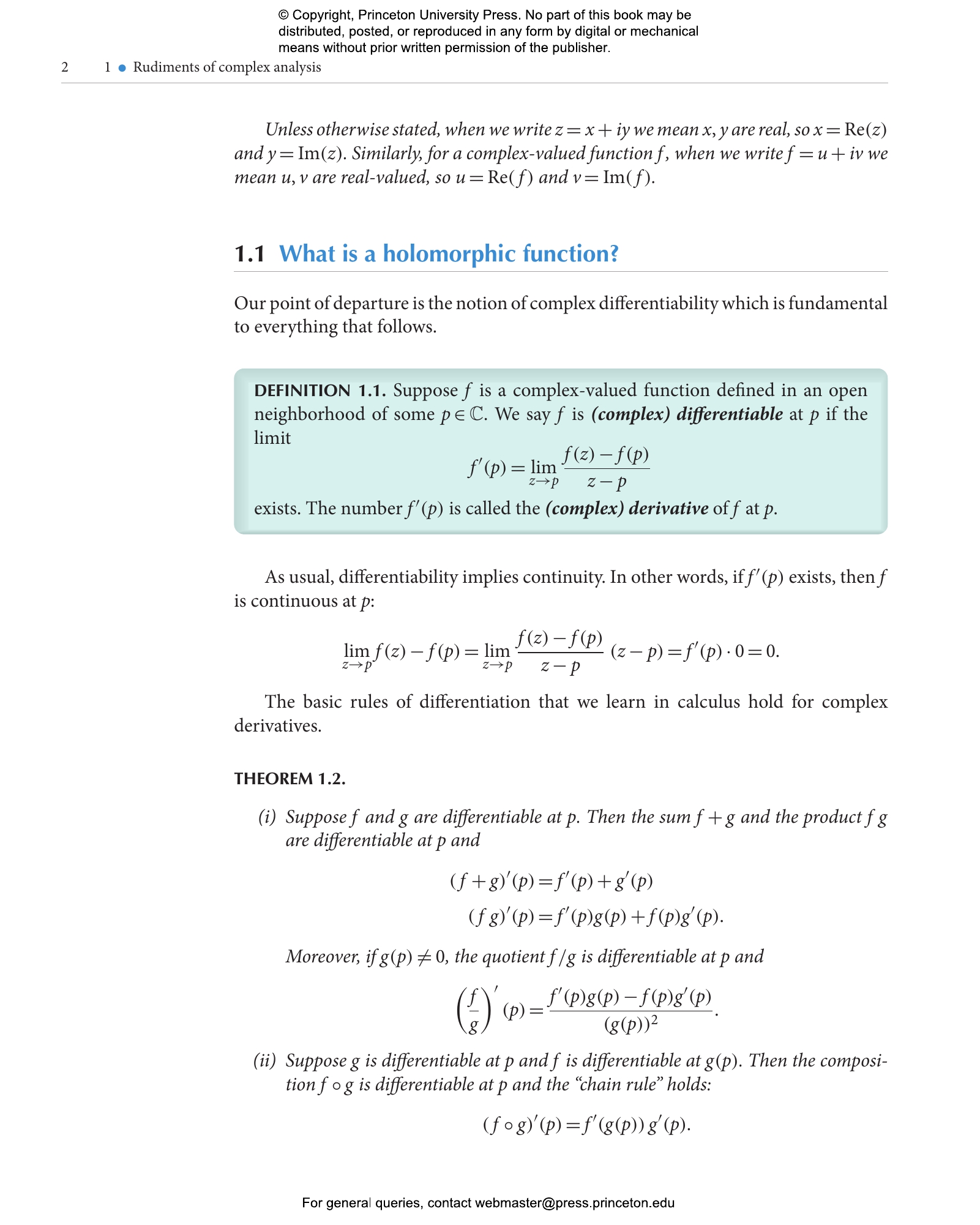
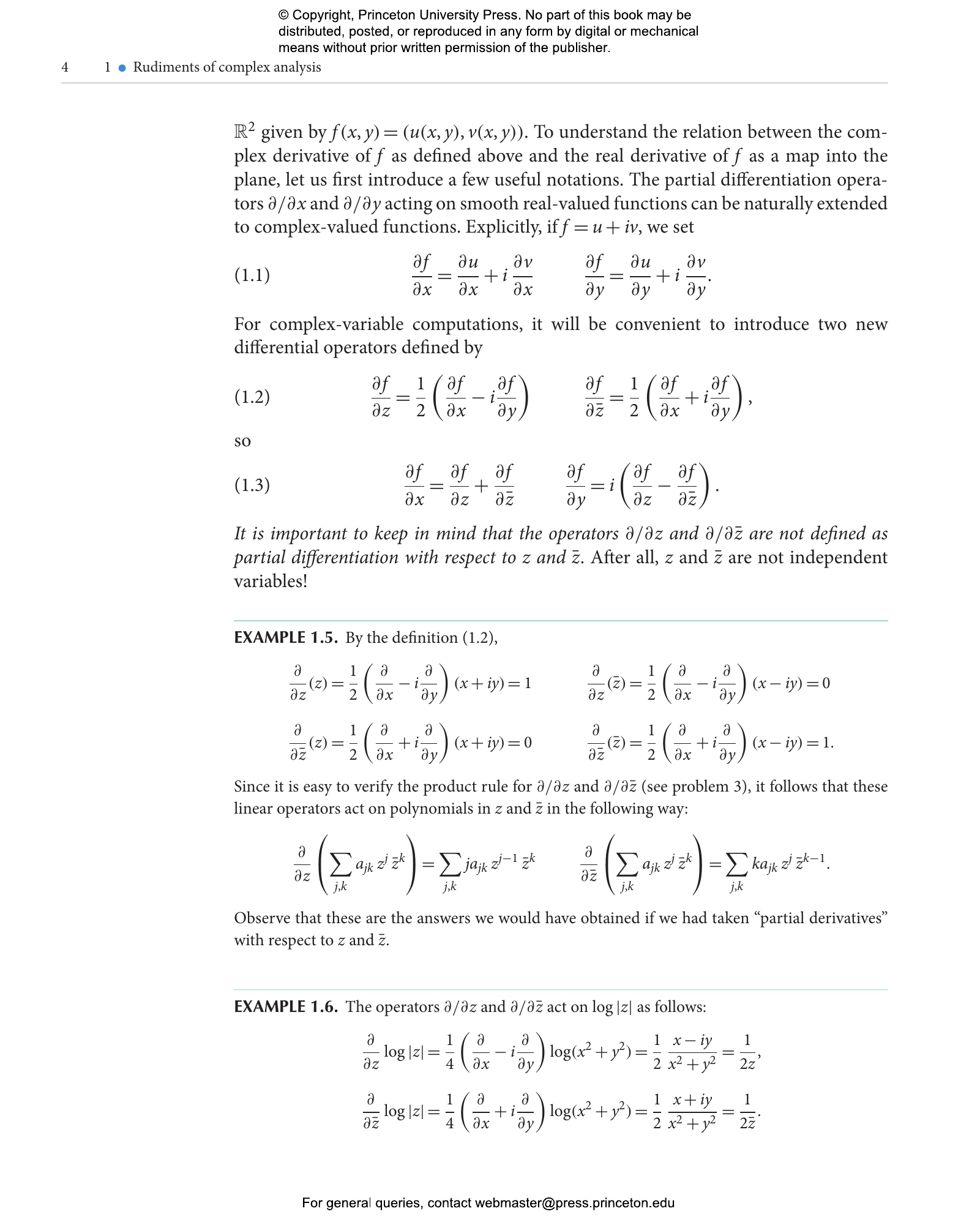
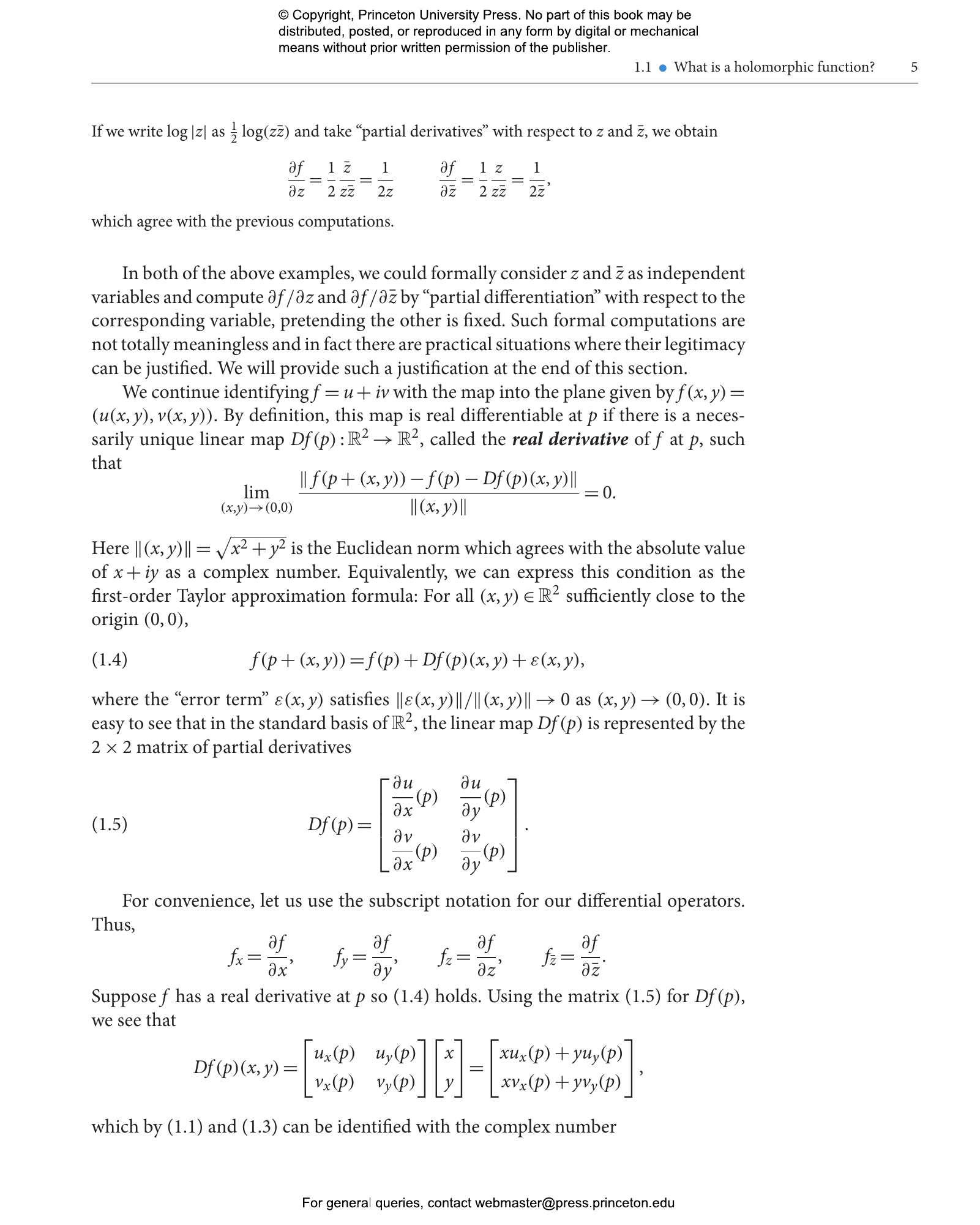
- 1.1 What is a holomorphic function?
- 1.2 Complex analytic functions
- 1.3 Complex integration
- 1.4 Cauchy’s theory in a disk
- 1.5 Mapping properties of holomorphic functions
- Problems
- Chapter 2. Topological aspects of Cauchy’s theory
- 2.1 Homotopy of curves
- 2.2 Covering properties of the exponential map
- 2.3 The winding number
- 2.4 Cycles and homology
- 2.5 The homology version of Cauchy’s theorem
- Problems
- Chapter 3. Meromorphic functions
- 3.1 Isolated singularities
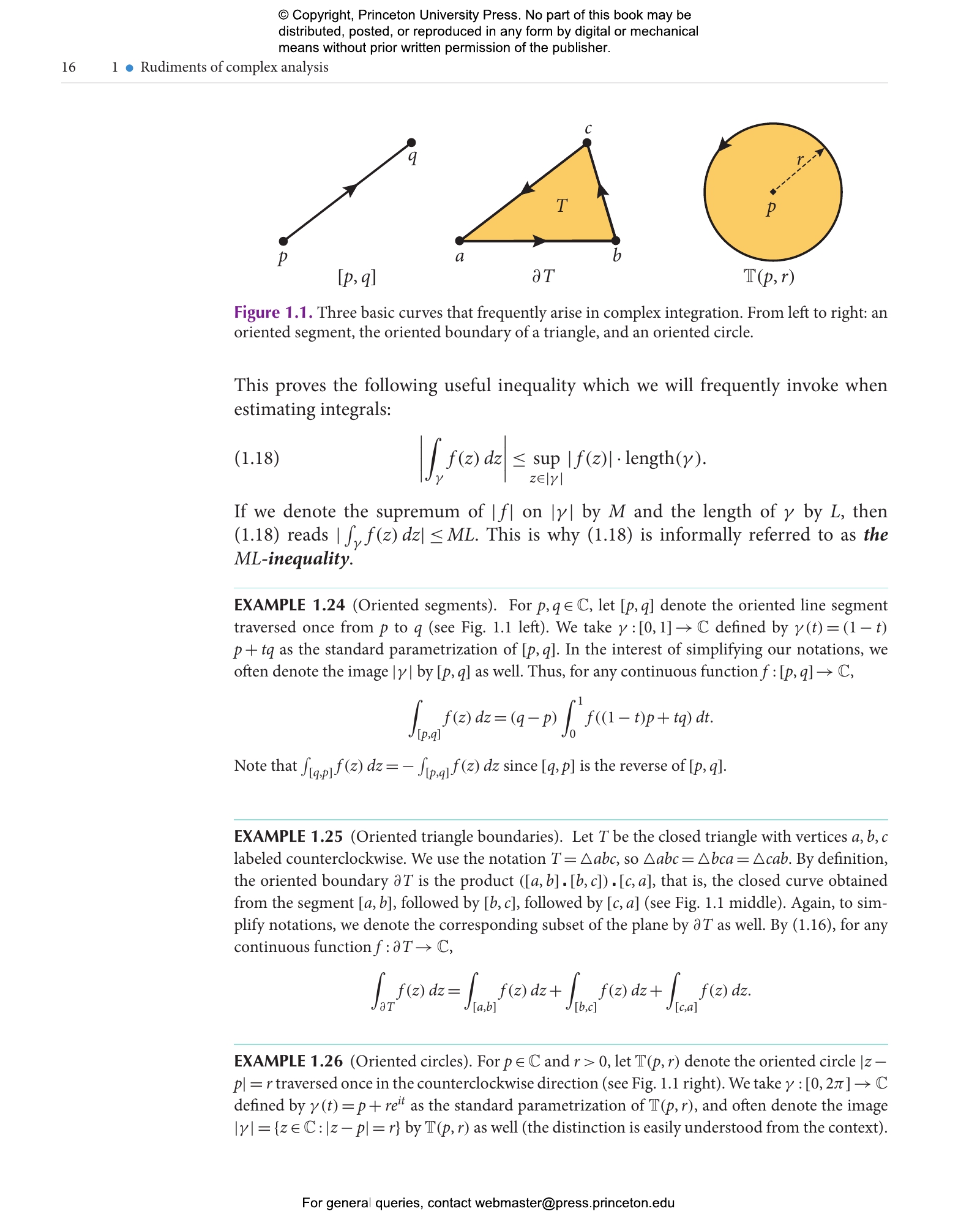
- 3.2 The Riemann sphere
- 3.3 Laurent series
- 3.4 Residues
- 3.5 The argument principle
- Problems
- Chapter 4. Möbius maps and the Schwarz lemma
- 4.1 The Möbius group
- 4.2 Three automorphism groups
- 4.3 Dynamics of Möbius maps
- 4.4 Conformal metrics
- 4.5 The hyperbolic metric
- Problems
- Chapter 5. Convergence and normality
- 5.1 Compact convergence
- 5.2 Convergence in the space of holomorphic functions
- 5.3 Normal families of meromorphic functions
- Problems
- Chapter 6. Conformal maps
- 6.1 The Riemann mapping theorem
- 6.2 Schlicht functions
- 6.3 Boundary behavior of Riemann maps
- Problems
- Chapter 7. Harmonic functions
- 7.1 Elementary properties of harmonic functions
- 7.2 Poisson’s formula in a disk
- 7.3 Some applications of Poisson’s formula
- 7.4 Boundary behavior of harmonic functions
- 7.5 Harmonic measure on the circle
- Problems
- Chapter 8. Zeros of holomorphic functions
- 8.1 Infinite products
- 8.2 Weierstrass’s theory of elementary factors
- 8.3 Jensen’s formula and its applications
- 8.4 Entire functions of finite order
- Problems
- Chapter 9. Interpolation and approximation theorems
- 9.1 Mittag-Leffler’s theorem
- 9.2 Elliptic functions
- 9.3 Rational approximation
- 9.4 Finitely connected domains
- Problems
- Chapter 10. The holomorphic extension problem
- 10.1 Regular and singular points
- 10.2 Analytic continuation
- 10.3 Analytic arcs and reflections
- 10.4 Two removability results
- Problems
- Chapter 11. Ranges of holomorphic functions
- 11.1 Bloch’s theorem
- 11.2 Picard’s theorems
- 11.3 A rescaling approach to Picard and Montel
- 11.4 Ahlfors’s generalization of the Schwarz-Pick lemma
- Problems
- Chapter 12. Holomorphic (branched) covering maps
- 12.1 Covering spaces
- 12.2 Holomorphic coverings and inverse branches
- 12.3 Proper maps and branched coverings
- 12.4 The Riemann-Hurwitz formula
- Problems
- Chapter 13. Uniformization of spherical domains
- 13.1 The modular group and thrice punctured spheres
- 13.2 The uniformization theorem
- 13.3 Hyperbolic domains
- 13.4 Conformal geometry of topological annuli
- Problems
- Bibliography
- Image credits
- Index
"A no-nonsense, clearly written graduate level textbook . . . . far more approachable than many other books on complex analysis"—Jonathan Shock, Mathemafrica
"An excellent textbook. . . . Carefully and precisely written in a lively style."—Ali Abkar, zbMATH Open
"Beautifully produced, beautifully written, on an incomparably beautiful area of mathematics, this is an inspirational book that I shall gratefully return to again and again."—Nick Lord, Mathematical Gazette
"This is a delightful book on complex analysis with a nice geometrical/topological flavor. It provides good foundations in the classical theory, and presents beautiful bridges between complex analysis and related subjects, such as topology and real analysis, as well as connections to more advanced topics, including Riemann surfaces and several complex variables."—John Milnor, Stony Brook University
"This wonderful book on complex analysis will certainly become a reference. In his masterful treatment of the subject, Zakeri balances the geometric and analytic viewpoints. The inclusion of recent developments and a wealth of historical notes makes the text even more attractive. I wish I had A Course in Complex Analysis when I was a student."—François Berteloot, Institut de Mathématiques de Toulouse
“Aimed at beginning graduate students, this book presents a streamlined and geometric approach to many of the highlights of complex analysis. Each chapter includes numerous examples, historical notes, attractive figures, and a wide range of interesting exercises. The breadth of material covered makes A Course in Complex Analysis an attractive addition to every mathematician’s library.”—Pamela Gorkin, Bucknell University
“Filled with interesting problems, A Course in Complex Analysis is a substantial textbook. Zakeri has invested a huge effort in its construction and arrangement and there is much to admire.”—Paul Martin, Colorado School of Mines
“Careful, consistent, and clear, this comprehensive textbook covers all the main topics of classical complex analysis and does so in admirable detail. The quality of the exposition and the breadth of material sets it apart. A lot of thought has gone into this book and any student of analysis will find it helpful.”—Tom Carroll, University College Cork